Computer Organization and Design 笔记 - Arithmetic for Computers

Addition and Subtraction

1. Add (add), and immediate (addi), and subtract (sub) cause exceptions on overflow. MIPS detects overflow with an exception (or interrupt ), which is an unscheduled procedure call. The address of current instruction is saved and the computer jumps to predefined address to invoke the appropriate routine for that exception.

   MIPS uses exception program counter (EPC) to contain the address of the instruction that causes the exception. The instruction move from system control (mfc0) is used to copy EPC into a general-purpose register.

2. Add unsigned (addu), add immediate unsigned (addiu), and subtract unsigned (subu) do not cause exceptions on overflow. Programmers can trap overflow anyway: when overflow occurs, the sign bit of the result is not properly set. Compairing with sign bits of operands, the sign bit of the result can be determined.

SIMD (single instruction, multiple data): By partitioning the carry chains within a 64-bit adder, a processor could perform simultaneous operations on a short vecters of eight 8-bit operands, four 16-bit operands, etc. Vectors and 8-bit data often appears in multimedia routine.

Multiplication

multiplicand * multiplier = product

Sequential Version of the Multiplication

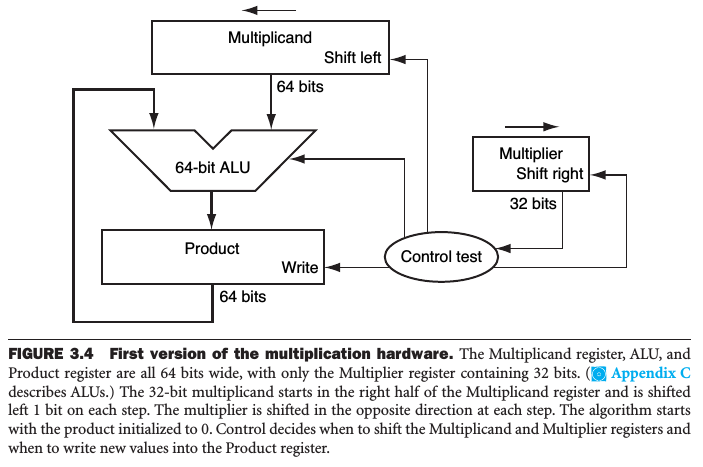
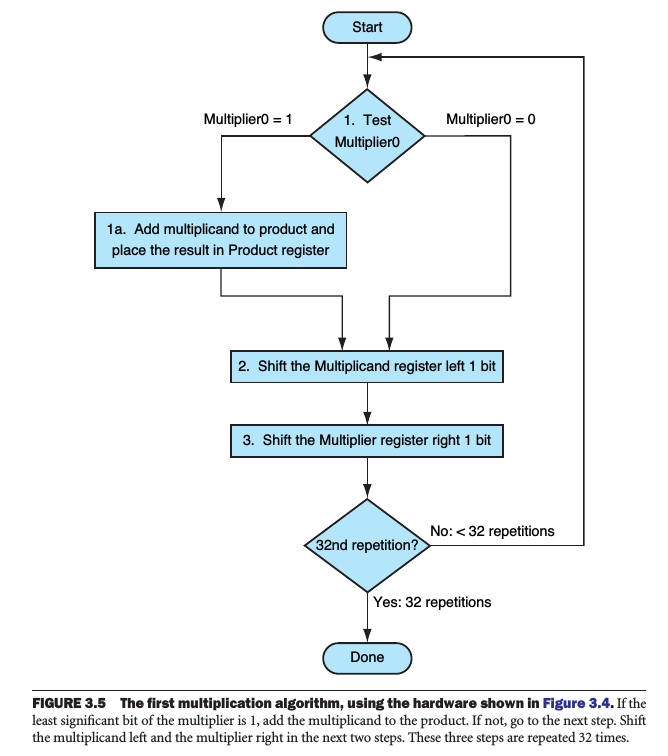
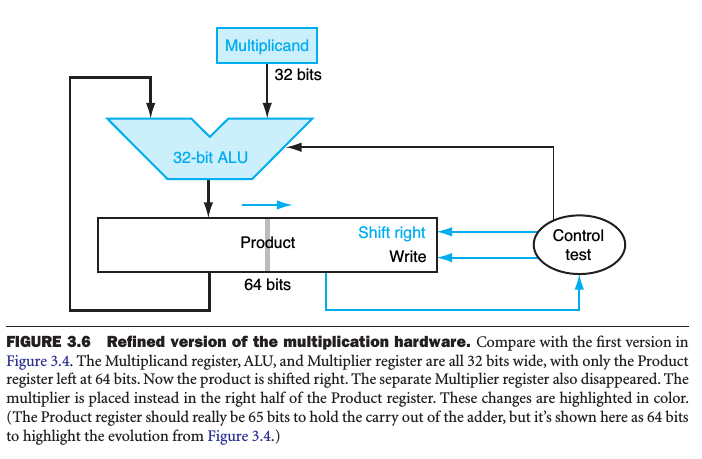
Refined version:

• Init: put multiplier to the left 32-bit of the product register.
• Cycle:
  1. if the last bit of product register is 1, add the left 32-bit with the multiplicand
  2. shift right the product register
• Final: the product register contains the 64-bit product

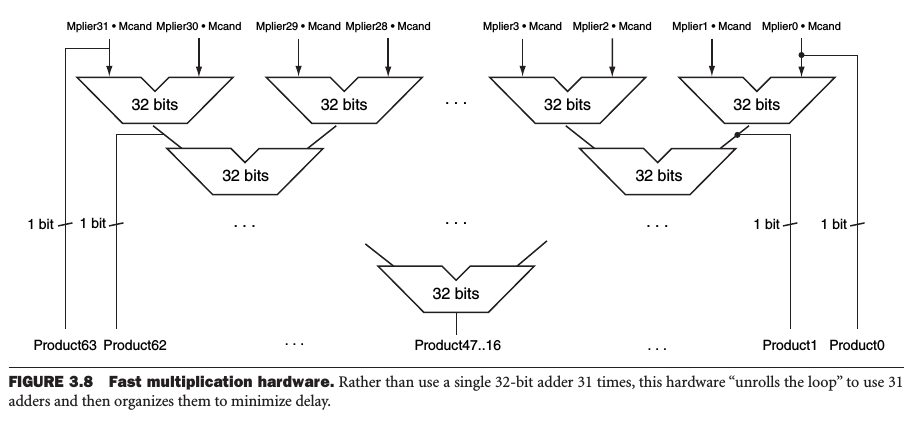
Faster Multiplication

A way to organize these 32 addtions is in a parallel tree:

Multiply in MIPS

The registers Hi and Lo contains the 64-bit product. Call mflo to fetch the 32-bit product, mfhi can be used to get Hi to test for overflow.

Division

Dividend = Quotient * Divisor + Remainder

Division Algorithm

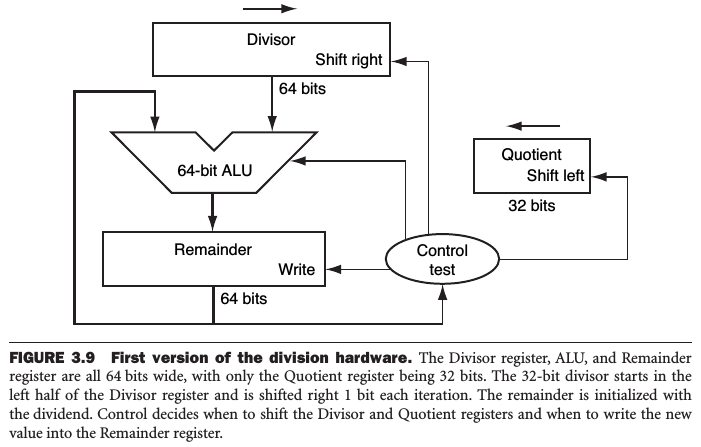
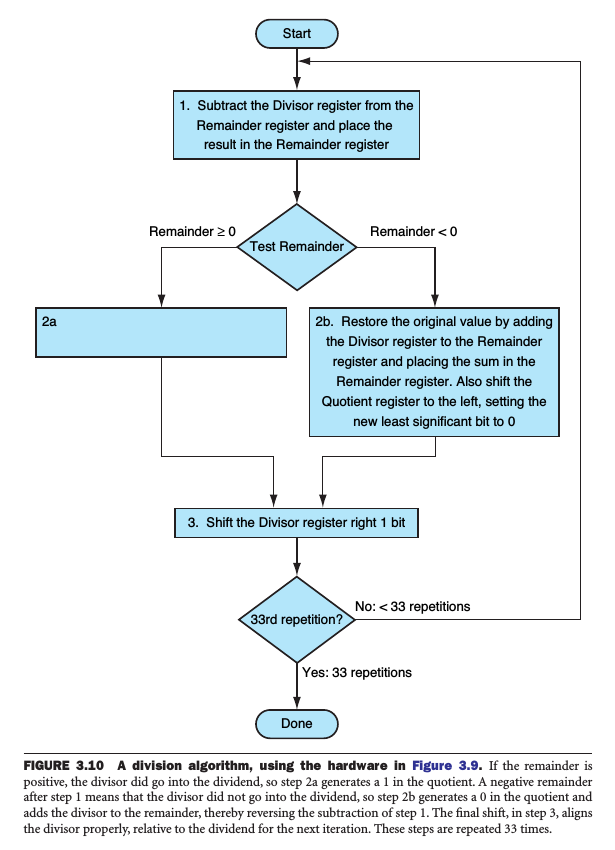
Improved version:

• Init: put the dividend in the right 32-bit of remainder register.
• Cycle:
  1. subtract the left 32-bit of remainder by the divisor
  2. shift left the remaider register
  3. set the last bit as new quotient bit
• Final: the left 32-bit contains the remainder, right 32-bit contains the quotient.

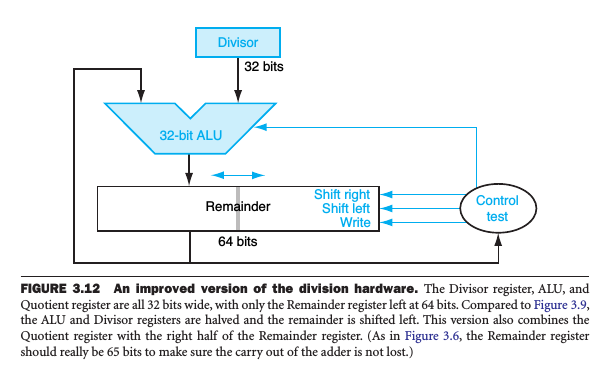
Faster Division

SRT division: try to guess several quotient bits per step, using a table lookup based on the upper bits of the dividend and remainder. The key is guessing the value to subtract.

Divide in MIPS

Hi contains the remainder, and Lo contains the quotient after the divide instruction complete.

MIPS divide instructions ignore overflow. MIPS software must check the divisor to discover division by 0 as well as overflow.

Floating Point

scientific notation A notation that renders numbers with a single digit to the left of the decimal point.

normalized A number in floating-point notation that has no leading 0s.

fraction The value, generally between 0 and 1, placed in the fraction field.

exponent In the numerical representation system of floating-point arithmetic, the value that is placed in the exponent field.

overflow the exponent is too large to be represented in the exponent field.

floating point Computer arithmetic that represents numbers in which the binary point is not fixed.

In general, floating-point numbers are of the form: $(-1)^S \times F \times 2^E$

MIPS float: sign(1 bit) + exponent(8 bit) + fraction(23 bit) MIPS double: s(1 bit) + exponent(11 bit) + fraction(52 bit)

IEEE 754 uses a bias of 127 for single precesion, and makes the leading 1 implicit. Since 0 has no leading 1, it's given the reserved exponent 0 so that hardware won't attach a leading 1.

Thus 00…00 represents 0; the representation of the rest are in the following form:

$(-1)^S \times (1 + Fraction)\times 2^(Exponent - Bias)$

The exponent is located left and the bias is for comparison convenience.

本文采用 知识共享署名 4.0 国际许可协议（CC-BY 4.0）进行许可，转载注明来源即可： https://harttle.land/2014/02/12/computer-design-arithmetic.html。如有疏漏、谬误、侵权请通过评论或 邮件 指出。